TUTORIALS
How to calculate quantity of steel in slab (circular shaped slab)

Let’s assume a circular slab. In what way the steel must be distributed? First of all, draw linear lines beginning from the diameter of the circle. There are eight lines from L1 to L8. L1 begins from the middle and diameter of the circle. The lengths from L1 to L8 will reduce gradually.
L1 is slab diameter.
Overall length of L2 = square root (R2 – (h1)2) x 2
Here h1 is distance amid L1 and L2.
L3 = square root (R2 – (h2)2) x 2
Here h2 is distance amid L1 and L3
And h3 is going to be the distance amid L1 and L4
L4 = square root (R2 – (h3)2) x 2
Once one half of the circle is measured, the lengths of L1 to L8 are measured and on having same diameter of bars and interval, multiply with 2 to obtain second half.
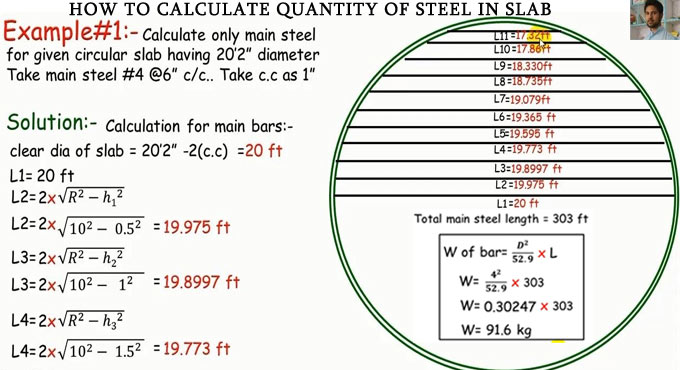
Instance A – Compute simply main steel for provided circular slab with 20’2” diameter. The main steel is number 4 @6” c/c. Assume 1” as concrete cover.
Resolution – Computation for main bars.
Slab clear diameter = 20’2” – 2(concrete cover) = 20’2”- 2(1) = 20 ft
L1 = 20 ft
L2 = square root (R2 – (h1)2) x 2 = square root ((10)2 – (0.5)2) x 2 = 19.975 ft
L3 = square root (R2 – (h2)2) x 2 = square root ((10)2 – (1)2) x 2 = 19.8997 ft
L4 = square root (R2 – (h3)2) x 2 = square root ((10)2 – (1.5)2) x 2 = 19.773 ft
Similarly, L5 = 19.595 ft
L6 = 19.365 ft
L7 = 19.079 ft
L8 = 18.735 ft
L9 = 18.330 ft
L10 = 17.86 ft
L11 = 17.32 ft
Overall main steel length = 303 ft (approx)
How much steel is needed?
Bar weight = D2/52.9 x Length = (4)2/52.9 x 303 = 0.30247 x 303 = 91.6 kg
To get more details, go through the following video tutorial.
Lecturer: SL Khan