TUTORIALS

How to Estimate Materials for RCC Dome

There are several types of domes. However, in this tutorial we will just do the computation of only spherical domes. Keep in mind, spherical dome is basically a half sphere. On cutting the full sphere in two equal parts, the one part is called a half sphere. Also, keep in mind, the dome may be simply a segmental sphere or part of half sphere.

The calculation for a half sphere is pretty simple; however the calculation for a segmented sphere is quite complicated.

Volume of hollow sphere = 4π (R³ – r³)/3

Where R represents external surface radius and r represents interior surface radius.

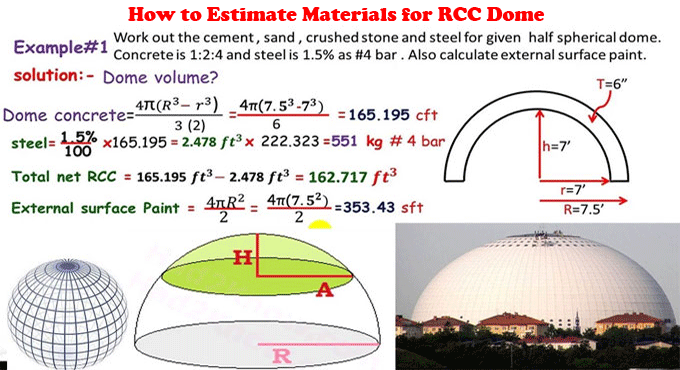
Now lets us calculate the cement, sand, crushed stone, external surface paint and steel for half spherical dome. Concrete is 1:2:4 and steel is 1.5% as #4 bar.

R = 7.5 feet and thickness ‘T’ = 6 inches,
r = 7 feet and height ‘h’ = 7 feet

Then, volume of half spherical dome = 4π (R³ – r³)/3(2) = 4π ((7.5)³ – (7)3)/6 = 165.195 cft
Steel = 1.5/100 x 165.195 = 2.478 ft³

Multiply it with unit weight of mild steel which provides, 2.478 ft³ x 222.323 kg/cft = 55 kg # 4 bar

Total net RCC = 165.195 ft³ – 2.478 ft³ = 162.717 ft³

External surface paint = 4πR²/2 (as the sphere is half) = 4π (7.5)²/2 = 353.43 ft²

Therefore, total PCC work (1:2:4) = 162.717 ft³

We know that material = ratio of material/sum of ratio x Dry volume

Then, cement = 1/7 x 162.717 x 1.54 = 35.8 ft³

Furthermore, cement (in bags) = 35.8/1.25 = 29 bags

Sand = 35.8 x 2 = 71.6 feet³

Crushed stone = 35.8 x 4 = 143.2 ft³

Let’s us take another example. There is a segmental dome with thickness ‘T’ = 6 inches, the height ‘h2 = 4.5 feet, radius of full sphere ‘R1’ = 10 feet, interior radius ‘R2’ = 9.5 feet and the external height h1 = 5 feet.

Therefore, dome volume = πh1² (3R1 – h1) / 3 – πh22 (3R2 – h²) /3

Dome volume = π (5)² (3 x 10 - 5) / 3 – π (4.5)² (3 x 9.5 – 4.5) / 3 = 145.56 ft³

Lecturer: SL Khan